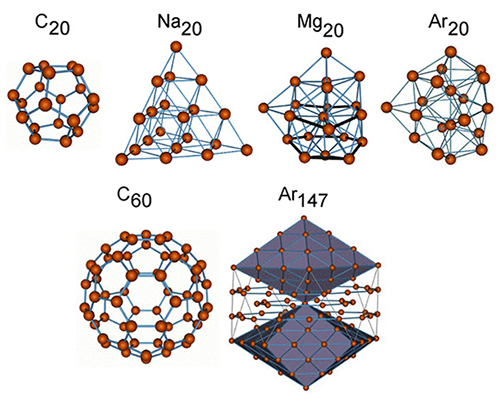
A group of atoms bound together by inter-atomic forces is called an atomic cluster. There is no qualitative distinction between small clusters and molecules. However, as the number of atoms in the system increases, atomic clusters acquire more and more specific properties making them unique physical objects different from both single molecules and from the solid-state.
In nature, there are many different types of atomic clusters: van der Waals clusters, metallic clusters, fullerenes, molecular, semiconductor, mixed clusters, and their shapes can depart considerably from the common spherical form: arborescent, linear, spirals, etc. Usually, one can distinguish between different types of clusters by the nature of the forces between the atoms, or by the principles of spatial organization within the clusters. Clusters can exist in all forms of matter: solid-state, liquid, gases and plasmas.
Figure 1. Different nature of forces between the atoms results in different principles of their organization within clusters and complex molecules.
In Figure 1, we present images of a few clusters in order to show a big variety of the cluster forms existing in nature. We also show the structure of the α/β-triosophosphate-isomerase globule aiming to stress that complex molecules such as proteins can be treated as clusters of subunits and that each of the subunits is a cluster on its own.
The novelty of atomic cluster physics arises mostly from the fact that cluster properties explain the transition from single atoms or molecules to the solid-state. Modern experimental techniques have made it possible to study this transition. By increasing the cluster size, one can observe the emergence of the physical features in the system, such as plasmon excitations, electron conduction band formation, superconductivity and superfluidity, phase transitions, fission and many more. Most of these many-body phenomena exist in solid-state but are absent for single atoms.
The science of clusters is a highly interdisciplinary field. Atomic clusters concern astrophysicists, atomic and molecular physicists, chemists, molecular biologists, solid-state physicists, nuclear physicists, plasma physicists, technologists all of whom see them as a branch of their subjects but cluster physics is a new subject in its own right.
Distinctive properties of atomic clusters: cluster magic numbers
Atomic clusters, as new physical objects, possess some properties, which are distinctive characteristics of these systems. The cluster geometry turns out to be an important feature of clusters, influencing their stability and vice-versa. The determination of the most stable cluster forms is not a trivial task and the solution of this problem is different for various types of cluster. The stability of clusters and the their transformations is a theme which does not exist at the atomic level and is not of great significance for solid-state but is of crucial importance for AC systems. This problem is closely connected to the problem of cluster magic numbers.
In Fig. 2, we present the mass spectra measured for Ar [H. Haberland (Ed.), Clusters of atoms and molecules, theory, experiment and clusters of atoms, Springer series in chemical physics, 52, Springer, Berlin, Heidelberg, New York (1994)] and Na [de Heer, Rev. Mod. Phys. 65, 611 (1993)] clusters, which clearly demonstrate the emergence of magic numbers (pronounced maxima in the spectra). The forces binding atoms in these two different types of clusters are different. The argon (noble gas) clusters are formed by van der Waals forces, while atoms in the sodium (alkali) clusters are bound by the delocalized valence electrons moving in the entire cluster volume. The differences in the inter-atomic potentials and pairing forces lead to the significant differences in structure between Na and Ar clusters, their mass spectra and their magic numbers.
Figure 2. Mass spectra measured for Ar and Na clusters. The intense peaks indicate enhanced stability.
In Fig. 3, we present and compare the geometries of a few small Na and Ar clusters of the same size [Solov'yov, Solov'yov, Greiner, (2002); Solov'yov, Solov'yov, Greiner, Koshelev, Shutovich (2003)]. It is clear from Fig. 3 that different principles of cluster organization result in different geometries of the alkali and noble gas cluster families.
Figure 3. Geometries and the point symmetry groups of some Na and Ar clusters calculated in [Solov'yov, Solov'yov, Greiner, (2002); Solov'yov, Solov'yov, Greiner, Koshelev, Shutovich (2003)].
Such differences can easily be explained. The van der Waals forces lead to enhanced stability of cluster geometries based on the most dense icosahedral packing. The most prominent peaks in mass spectra of argon clusters correspond to completed icosahedral shells of 13, 55, 147, 309, etc. atoms. The origin of these magic numbers can be understood on the basis of the classical equations. The origin of the sodium cluster magic numbers is different and is based on the principles of quantum mechanics. In this case the cluster magic numbers 8, 20, 34, 40, 58, 92, etc. correspond to the completed shells of the delocalised electrons: 1s21p61d102s21f142p6, etc. This feature of small metal clusters make them qualitatively similar to atomic nuclei for which quantum shell effects play the crucial role in determining their properties [Eisenberg, Greiner, Nuclear theory, North Holland, Amsterdam (1987)].
Electron excitations in atomic clusters
Electron excitations in metal cluster systems have a profoundly collective nature [Bréchignac, Connerade, J. Phys. B 27, 3795 (1994)]. They can be pictured as oscillations of electron density against ions, the so-called plasmon oscillations. This name is carried over from solid-state physics where a similar phenomenon occurs. Collective electron excitations have also been studied for single atoms and molecules. In this case the effect is known under the name of the shape or the giant resonance. The name giant resonance came to atomic physics from nuclear physics, where the collective oscillations of neutrons against protons have been investigated [Eisenberg, Greiner, Nuclear theory, North Holland, Amsterdam (1987)].
The interest of plasmon excitations in small metal clusters is connected with the fact that the plasmon resonances carry a lot of useful information about cluster electronic and ionic structure. By observing plasmon excitations in clusters one can study, for example, the transition from the pure classical Mie picture of the plasmon oscillations to its quantum limit or to detect cluster deformations by the value of splitting of the plasmon resonance frequencies. The plasmon resonances can be seen in the cross sections of various collision processes: photoabsorption and photoionization, electron inelastic scattering, electron attachment, bremsstrahlung [C. Guet, P. Hobza, F. Spiegelman, F. David (Eds.), NATO advanced study institute, session LXXIII, summer school "Atomic clusters and nanopartides", Les Houches, France, July 2–28, 2000, EDP Sciences and Springer Verlag, Berlin, Heidelberg, New York, Hong Kong, London, Milan, Paris, Tokyo (2001)].
In Fig. 4, we present experimentally measured and theoretically calculated cross section for the photoabsorption of some Na and Mg clusters [Solov'yov, Solov'yov, Greiner, (2004)]. The cross sections are resonantly enhanced owing to the excitation of plasmon oscillations in the target cluster.
Figure 4. Photoabsortion spectra of some Na and Mg clusters.
Fusion process of atomic clusters
The formation of a sequence of cluster magic numbers should be closely connected to the mechanisms of cluster formation and growth. It is natural to expect that one can explain the magic numbers sequence and find the most stable cluster isomers by modelling mechanisms of cluster assembly and growth, i.e. the fusion process of atomic clusters [Solov'yov, Solov'yov, Greiner, Koshelev, Shutovich (2003); Solov'yov, Solov'yov, Greiner, (2004)].
In the recent work [Solov'yov, Solov'yov, Greiner, Koshelev, Shutovich (2003); Solov'yov, Solov'yov, Greiner, (2004)], the fusion algorithm was formulated in a most simple, but general form. In the most simple scenario, it was assumed that atoms in a cluster are bound by Lennard-Jonnes potentials and the cluster fusion takes place atom by atom. In this process, new atoms are placed on the cluster surface in the middle of the cluster faces. Then, all atoms in the system are allowed to move, while the energy of the system is decreased. The motion of the atoms is stopped, when the energy minimum is reached. The geometries and energies of all cluster isomers found in this way are stored and analysed. The most stable cluster configuration (cluster isomer) is then used as a starting configuration for the next step of the cluster growing process.
Starting from the initial tetrahedral cluster configuration and using the strategy described above, the cluster fusion paths have been analysed up to the cluster sizes of more than 150 atoms. We have found that in this way practically all known global minimum structures of the Lennard-Jonnes clusters can be determined. Fig. 5 shows the images of the Lennard-Jonnes global energy minimum cluster isomers [Solov'yov, Solov'yov, Greiner, (2004)]. The mass numbers of the represented clusters correspond to the magic numbers of the noble gas (Ar, Kr, Xe) clusters.
Figure 5. Images of the Lennard-Jonnes global energy minimum cluster isomers. The mass numbers of the pictured clusters correspond to the magic numbers of the noble gas (Ar, Kr, Xe) clusters.